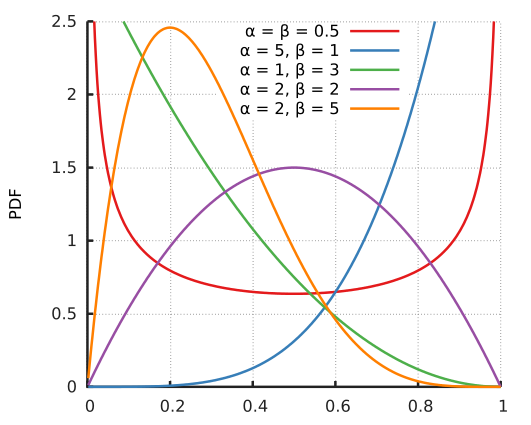
The beta distribution
The parameters are \(\alpha,\beta>0\), and \(A,B\) with \(B\ge A\).
\begin{equation*}
f(x;\alpha,\beta,A,B)=\frac{1}{B-A}\frac{\Gamma(\alpha+\beta)}{\Gamma(\alpha)\Gamma(\beta)}\left(\frac{x-A}{B-A}\right)^{\alpha-1}\left(\frac{B-x}{B-A}\right)^{\beta-1}
\end{equation*}
For \(A\le x\le B\)
When \(A=0,B=1\), it is called the standard Beta distribution.
\begin{equation*}
\mu=A+(B-A)\frac{\alpha}{\alpha+\beta}
\end{equation*}
\begin{equation*}
\sigma^{2}=\frac{(B-A)^{2}\alpha\beta}{(\alpha+\beta)^{2}(\alpha+\beta+1)}
\end{equation*}
Essentially, \(A,B\) are used to stretch/shift the curve. It is defined only for values between \(A\) and \(B\).
It is often used to model the proportion of percentage of a quantity in different samples (e.g. % of 24-hour day an individual is asleep).